UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreTrigonometry (originated from Greek word trigonon means triangle and metron means measure) is a branch of mathematics which deals with the study of relationship involving length of sides and angles of triangles.
Trigonometric Ratios:
Consider a right-angled triangle with a corresponding angle \(\theta\).
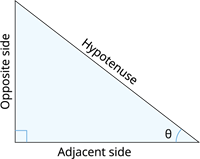
In the figure, the side opposite to the given angle \(\theta\) is called the 'Opposite side', the side adjacent to the given angle \(\theta\) is called the 'Adjacent side', and the greatest side opposite to right angle is called the 'hypotenuse'.
Important!
Click here! To recall the Pythagoras theorem. (Since we frequently make use of the right-angled triangle.)
Based on the given corresponding angle \(\theta\), we will learn about three basic trigonometric ratios satisfied by a right-angled triangle.
Each of these ratios is obtained by dividing one side of the right-angled triangle by another.
The three basic trigonometric ratios are:
- Sine
- Cosine
- Tangent
The table below depicts the relation of these ratios with the right-angled triangle.
| Name of the angle | Sine | Cosine | Tangent |
| Short form of the angle | \(\sin\) | \(\cos\) | \(\tan\) |
| Measurements related to the right-angled triangle |  |  |  |
| Relationship | \(\sin \theta\) \(=\) \(\frac{\text{Opposite side}}{\text{Hypotenuse}}\) | \(\cos \theta\) \(=\) \(\frac{\text{Adjacent side}}{\text{Hypotenuse}}\) | \(\tan \theta\) \(=\) \(\frac{\text{Opposite side}}{\text{Adjacent side}}\) |
Important!
1. The trigonometric ratios are unitless as they are defined in terms of ratios of sides of the triangle.
2. The ratios \(\sin \theta\), \(\cos \theta\) and \(\tan \theta\) should not be treated as \((\sin) \times (\theta)\), \((\cos) \times (\theta)\) and \((\tan) \times (\theta)\).
Register for free to see more content