PDF chapter test TRY NOW
Consider an equilateral triangle ABC with sides measuring 2 units.
That is AB = BC = CA = 2 units.
Draw a bisector of \angle A such that it meets BC at D.
The angle bisector of an equilateral triangle also bisects the side opposite it.
So, BD = DC = 1 unit.
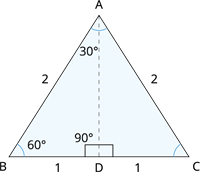
First, let us calculate the measure of angle bisector BD in the figure.
Consider the triangle ABD.
Since the given triangle is a right-angled triangle by the Pythagoras theorem, we have:
In a right angled triangle, \text{Hypotenuse}^{2} = \text{Adjacent side}^{2} + \text{Opposite side}^{2}.
AB^2 = BD^2 + DA^2.
DA^2 = AB^2 - BD^2
DA^2 = 2^2 - 1^2
DA^2 = 4 - 1
DA^2 = 3
\Rightarrow DA = \sqrt{3}
Therefore, for the considered right-angled triangle, we have:
| With respect to 30^{\circ} | With respect to 60^{\circ} |
| Opposite side = 1 units | Opposite side = \sqrt{3} units |
| Adjacent side = \sqrt{3} units | Adjacent side = 1 units |
| Hypotenuse = 2 units | Hypotenuse = 2 units |
Now, let us determine all the trigonometric ratios of 30^{\circ} and 60^{\circ}.
- Sine:
\sin 30^{\circ} | \sin 60^{\circ} |
\sin 30^{\circ} = \frac{\text{Opposite side}}{\text{Hypotenuse}} = \frac{1}{2} | \sin 60^{\circ} = \frac{\text{Opposite side}}{\text{Hypotenuse}} = \frac{\sqrt{3}}{2} |
- Cosine 30^{\circ}:
\cos 30^{\circ} | \cos 60^{\circ} |
\cos 30^{\circ} = \frac{\text{Adjacent side}}{\text{Hypotenuse}} = \frac{\sqrt{3}}{2} | \cos 60^{\circ} = \frac{\text{Adjacent side}}{\text{Hypotenuse}} = \frac{1}{2} |
- Tangent:
\tan 30^{\circ} | \tan 60^{\circ} |
\tan 30^{\circ} = \frac{\text{Opposite side}}{\text{Adjacent side}} = \frac{1}{\sqrt{3}} | \tan 60^{\circ} = \frac{\text{Opposite side}}{\text{Adjacent side}} = \frac{\sqrt{3}}{1} = \sqrt{3} |
Using these basic trigonometric ratios determine their reciprocals as follows:
- Cosecant:
\text{cosec}\,30^{\circ} | \text{cosec}\,60^{\circ} |
\text{cosec}\,30^{\circ} = \frac{1}{\sin 30^{\circ}} = \frac{2}{1} = 2 | \text{cosec}\,60^{\circ} = \frac{1}{\sin 60^{\circ}} = \frac{2}{\sqrt{3}} |
- Secant:
\sec 30^{\circ} | \sec 60^{\circ} |
\sec 30^{\circ} = \frac{1}{\cos 30^{\circ}} = \frac{2}{\sqrt{3}} | \sec 60^{\circ} = \frac{1}{\cos 60^{\circ}} = \frac{2}{1} = 2 |
- Cotangent:
\cot 30^{\circ} | \cot 60^{\circ} |
\cot 30^{\circ} = \frac{\text{1}}{\tan 30^{\circ}} = \frac{\sqrt{3}}{1} = \sqrt{3} | \cot 60^{\circ} = \frac{\text{1}}{\tan 60^{\circ}} = \frac{1}{\sqrt{3}} |
Let us summarize all the trigonometric ratios of 30^{\circ} and 60^{\circ} in the following table.
\sin \theta | \cos \theta | \tan \theta | \text{cosec}\,\theta | \sec \theta | \cot \theta | |
\theta = 30^{\circ} | \frac{1}{2} | \frac{\sqrt{3}}{2} | \frac{1}{\sqrt{3}} | 2 | \frac{2}{\sqrt{3}} | \sqrt{3} |
\theta = 60^{\circ} | \frac{\sqrt{3}}{2} | \frac{1}{2} | \sqrt{3} | \frac{2}{\sqrt{3}} | 2 | \frac{1}{\sqrt{3}} |
