UPSKILL MATH PLUS
Learn Mathematics through our AI based learning portal with the support of our Academic Experts!
Learn moreWe will derive the trigonometric ratios of \(90^{\circ}\) with the help of a unit circle.
A circle with radius \(1\) unit centred at the origin is called a unit circle.
Here \(OQ\) \(=\) \(OP\) \(=\) \(OC\) \(=\) \(1\) unit (Radius).
Let us consider the first quadrant.
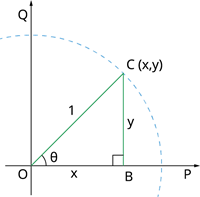
Let the point \(C(x,y)\) be any point on the unit circle and \(\angle COB\) \(=\) \(\theta\).
In the right angles triangle \(COB\) we have:
Opposite side \(=\) \(y\)
Adjacent side \(=\) \(x\)
Hypotenuse \(=\) \(1\)
Now, let us determine the trigonometric ratios in the first quadrant with the coordinate \(C\).
- Sine \(\theta\):
\(\sin \theta\) \(=\) \(\frac{\text{Opposite side}}{\text{Hypotenuse}}\)
\(=\) \(\frac{y}{1}\)
\(=\) \(y\)
- Cosine \(\theta\):
\(\cos \theta\) \(=\) \(\frac{\text{Adjacent side}}{\text{Hypotenuse}}\)
\(=\) \(\frac{x}{1}\)
\(=\) \(x\)
- Tangent \(\theta\):
\(\tan \theta\) \(=\) \(\frac{\text{Opposite side}}{\text{Adjacent side}}\)
\(=\) \(\frac{y}{x}\)
When \(\theta = 90^{\circ}\), \(OC\) coincides with \(OQ\) then \(Q = (0,1)\) where \(x\) \(=\) \(0\) and \(y\) \(=\) \(1\).
Then the trigonometric ratios are given by:
- Sine \(90^{\circ}\):
\(\sin 0^{\circ}\) \(=\) \(y\)
\(=\) \(1\)
- Cosine \(90^{\circ}\):
\(\cos 90^{\circ}\) \(=\) \(x\)
\(=\) \(0\)
- Tangent \(90^{\circ}\):
\(\tan 90^{\circ}\) \(=\) \(\frac{1}{0}\)
\(=\) not defined
Using these basic trigonometric ratios determine their reciprocals as follows:
- Cosecant \(90^{\circ}\):
\(\text{cosec}\,90^{\circ}\) \(=\) \(\frac{1}{\sin 90^{\circ}}\)
\(=\) \(\frac{1}{1}\)
\(=\) \(1\)
- Secant \(90^{\circ}\):
\(\sec 90^{\circ}\) \(=\) \(\frac{1}{\cos 90^{\circ}}\)
\(=\) \(\frac{1}{0}\)
\(=\) not defined
- Cotangent \(90^{\circ}\):
\(\cot 90^{\circ}\) \(=\) \(\frac{1}{\tan 90^{\circ}}\)
\(=\) \(\frac{0}{1}\)
\(=\) \(0\)
Let us summarize all the trigonometric ratios of \(90^{\circ}\) in the following table.
\(\sin \theta\) | \(\cos \theta\) | \(\tan \theta\) | \(\text{cosec}\,\theta\) | \(\sec \theta\) | \(\cot \theta\) | |
\(\theta = 90^{\circ}\) | \(1\) | \(0\) | not defined | \(1\) | not defined | \(0\) |
Register for free to see more content